One of the greatest privileges of working directly with the worldŌĆÖs most powerful quantum computer at ę╣╔½ų▒▓ź is building meaningful experiments that convert theory into practice. The privilege becomes even more compelling when considering that our current quantum processor ŌĆō our H2 system ŌĆō will soon be enhanced by Helios, a quantum computer potentially a stunning trillion times more powerful, and due for launch in just a few months. The moment has now arrived when we can build a timeline for applications that quantum computing professionals have anticipated for decades and which are experimentally supported.
ę╣╔½ų▒▓źŌĆÖs applied algorithms team has released an end-to-end implementation of a quantum algorithm to solve a central problem in knot theory. Along with an efficiently verifiable benchmark for quantum processors, it allows for concrete resource estimates for quantum advantage in the near-term. The research team, included ę╣╔½ų▒▓ź researchers Enrico Rinaldi, Chris Self, Eli Chertkov, Matthew DeCross, David Hayes, Brian Neyenhuis, Marcello Benedetti, and Tuomas Laakkonen of the Massachusetts Institute of Technology. In this article, Konstantinos Meichanetzidis, a team leader from ę╣╔½ų▒▓źŌĆÖs AI group who led the project, writes about the problem being addressed and how the team, adopting an aggressively practical mindset, quantified the resources required for quantum advantage:
Knot theory is a field of mathematics called ŌĆślow-dimensional topologyŌĆÖ, with a rich history, stemming from a wild idea proposed by Lord Kelvin, who conjectured that chemical elements are different knots formed by vortices in the aether. Of course, we know today that the aether theory was falsified by the Michelson-Morley experiment, but mathematicians have been classifying, tabulating, and studying knots ever since. Regarding applications, the pure mathematics of knots can find their way into cryptography, but knot theory is also intrinsically related to many aspects of the natural sciences. For example, it naturally shows up in certain spin models in statistical mechanics, when one studies thermodynamic quantities, and the magnetohydrodynamical properties of knotted magnetic fields on the surface of the sun are an important indicator of solar activity, to name a few examples. Remarkably, physical properties of knots are important in understanding the stability of macromolecular structures. This is highlighted by work of Cozzarelli and Sumners in the 1980ŌĆÖs, on the topology of DNA, particularly how it forms knots and supercoils. Their interdisciplinary research helped explain how enzymes untangle and manage DNA topology, crucial for replication and transcription, laying the foundation for using mathematical models to predict and manipulate DNA behavior, with broad implications in drug development and synthetic biology. Serendipitously, this work was carried out during the same decade as Richard Feynman, David Deutsch, and Yuri Manin formed the first ideas for a quantum computer.
Most importantly for our context, knot theory has fundamental connections to quantum computation, originally outlined by WittenŌĆÖs work in topological quantum field theory, concerning spacetimes without any notion of distance but only shape. In fact, this connection formed the very motivation for attempting to build topological quantum computers, where anyons ŌĆō exotic quasiparticles that live in two-dimensional materials ŌĆō are braided to perform quantum gates. The relation between knot theory and quantum physics is the most beautiful and bizarre facts you have never heard of.
The fundamental problem in knot theory is distinguishing knots, or more generally, links. To this end, mathematicians have defined link invariants, which serve as ŌĆśfingerprintsŌĆÖ of a link. As there are many equivalent representations of the same link, an invariant, by definition, is the same for all of them. If the invariant is different for two links then they are not equivalent. The specific invariant our team focused on is the Jones polynomial.
ŌĆŹ
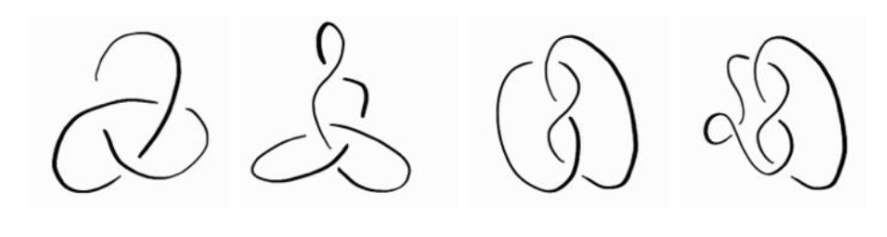
They all have the same Jones polynomial, as it is an invariant.
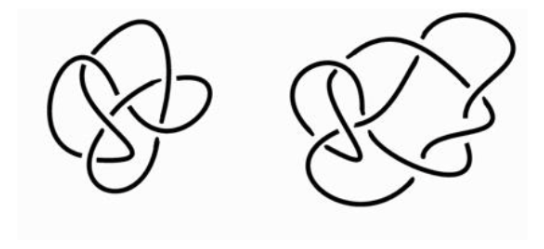
The mind-blowing fact here is that any quantum computation corresponds to evaluating the Jones polynomial of some link, as shown by the works of Freedman, Larsen, Kitaev, Wang, Shor, Arad, and Aharonov. It reveals that this abstract mathematical problem is truly quantum native. In particular, the problem our team tackled was estimating the value of the Jones polynomial at the 5th root of unity. This is a well-studied case due to its relation to the infamous Fibonacci anyons, whose braiding is capable of universal quantum computation.
Building and improving on the work of Shor, Aharonov, Landau, Jones, and Kauffman, our team developed an efficient quantum algorithm that works end-to end. That is, given a link, it outputs a highly optimized quantum circuit that is readily executable on our processors and estimates the desired quantity. Furthermore, our team designed problem-tailored error detection and error mitigation strategies to achieve a higher accuracy.
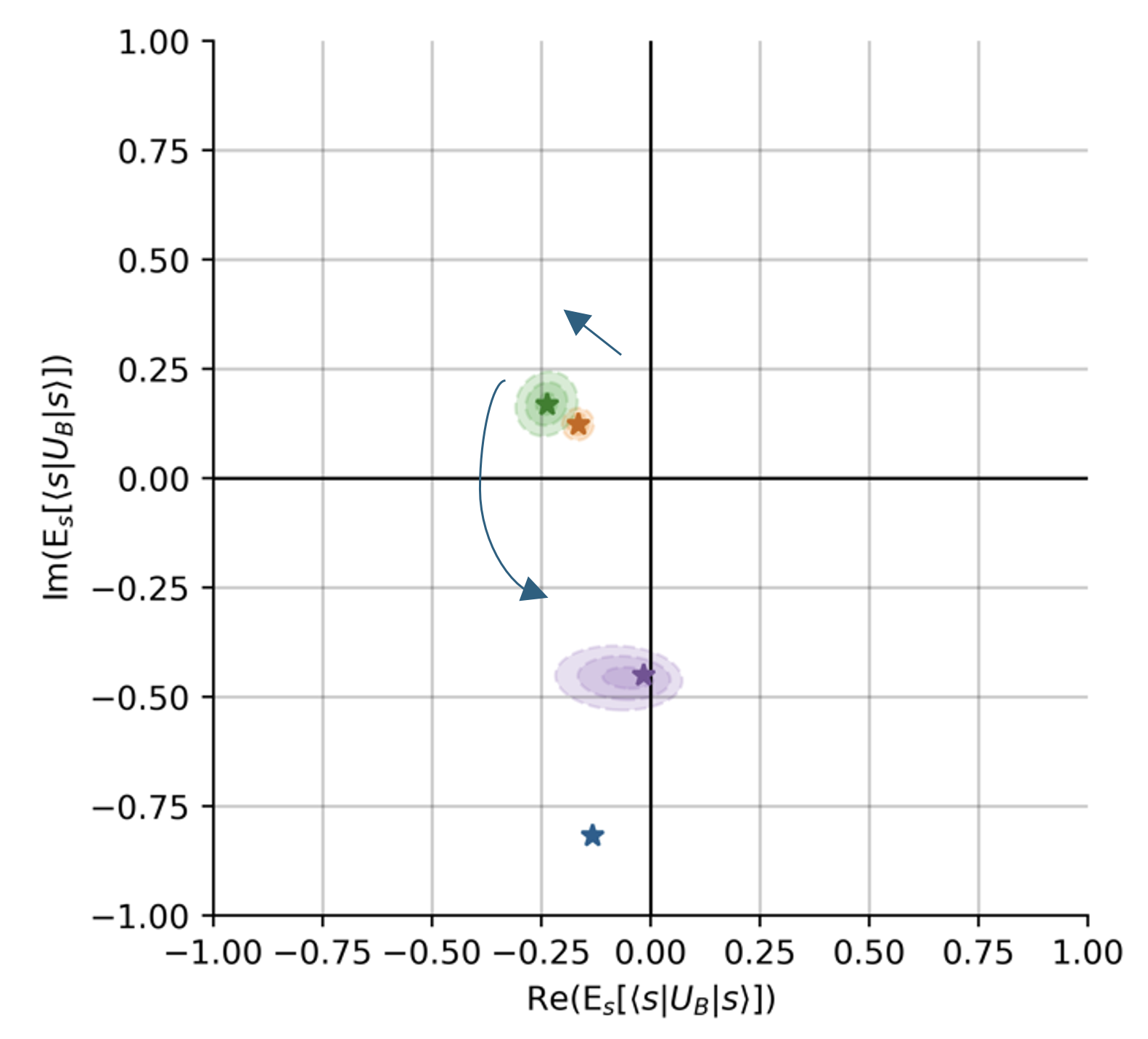
In addition to providing a full pipeline for solving this problem, a major aspect of this work was to use the fact that the Jones polynomial is an invariant to introduce a benchmark for noisy quantum computers. Most importantly, this benchmark is efficiently verifiable, a rare property since for most applications, exponentially costly classical computations are necessary for verification. Given a link whose Jones polynomial is known, the benchmark constructs a large set of topologically equivalent links of varying sizes. In turn, these result in a set of circuits of varying numbers of qubits and gates, all of which should return the same answer. Thus, one can characterize the effect of noise present in a given quantum computer by quantifying the deviation of its output from the known result.
The benchmark introduced in this work allows one to identify the link sizes for which there is exponential quantum advantage in terms of time to solution against the state-of-the-art classical methods. These resource estimates indicate our next processor, Helios, with 96 qubits and at least 99.95% two-qubit gate-fidelity, is extremely close to meeting these requirements. Furthermore, ę╣╔½ų▒▓źŌĆÖs hardware roadmap includes even more powerful machines that will come online by the end of the decade. Notably, an advantage in energy consumption emerges for even smaller link sizes. Meanwhile, our teams aim to continue reducing errors through improvements in both hardware and software, thereby moving deeper into quantum advantage territory.
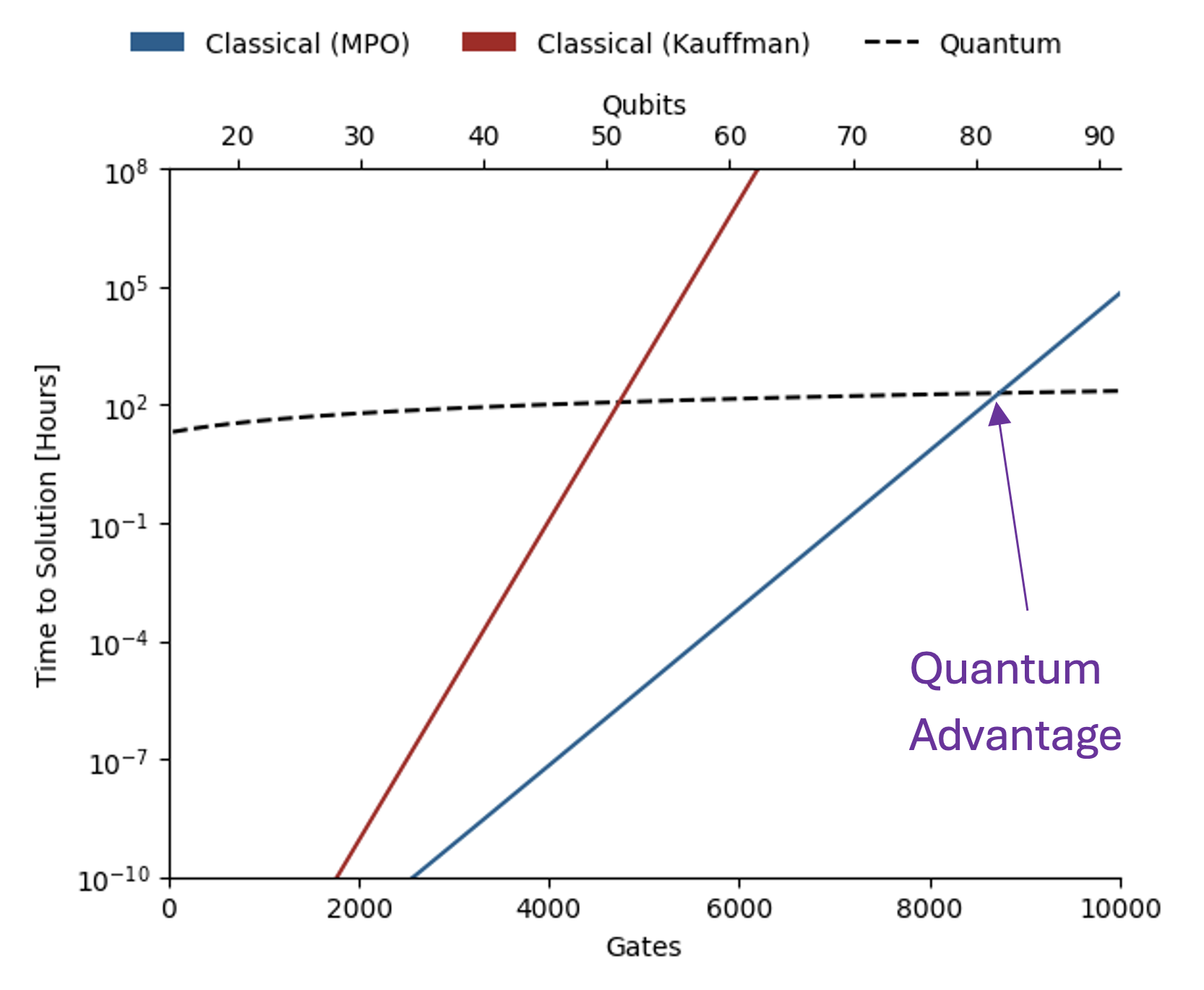
The importance of this work, indeed the uniqueness of this work in the quantum computing sector, is its practical end-to-end approach. The advantage-hunting strategies introduced are transferable to other ŌĆ£quantum-easy classically-hardŌĆØ problems. Our teamŌĆÖs efforts motivate shifting the focus toward specific problem instances rather than broad problem classes, promoting an engineering-oriented approach to identifying quantum advantage. This involves first carefully considering how quantum advantage should be defined and quantified, thereby setting a high standard for quantum advantage in scientific and mathematical domains. And thus, making sure we instill confidence in our customers and partners.
Edited



