The most common question in the public discourse around quantum computers has been, “When will they be useful?” We have an answer.
Very recently in Nature we a successful demonstration of a quantum computer generating certifiable randomness, a critical underpinning of our modern digital infrastructure. We explained how we will be taking a product to market this year, based on that advance – one that could only be achieved because we have the world’s most powerful quantum computer.
Today, we have made another huge leap in a different domain, providing fresh evidence that our quantum computers are the best in the world. In this case, we have shown that our quantum computers can be a useful tool for advancing scientific discovery.
Understanding magnetism
Our latest shows how our quantum computer rivals the best classical approaches in expanding our understanding of magnetism. This provides an entry point that could lead directly to innovations in fields from biochemistry, to defense, to new materials. These are tangible and meaningful advances that will deliver real world impact.
To achieve this, we partnered with researchers from Caltech, Fermioniq, EPFL, and the Technical University of Munich. The team used “π…´÷±≤•‚Äôs System Model H2 to simulate quantum magnetism at a scale and level of accuracy that pushes the boundaries of what we know to be possible.
As the authors of the paper state:
“We believe the quantum data provided by System Model H2 should be regarded as complementary to classical numerical methods, and is arguably the most convincing standard to which they should be compared.”
Our computer simulated the quantum Ising model, a model for quantum magnetism that describes a set of magnets (physicists call them ‚Äòspins‚Äô) on a lattice that can point up or down, and prefer to point the same way as their neighbors. The model is inherently ‚Äúquantum‚Äù because the spins can move between up and down configurations by a process known as ‚Äúquantum tunneling‚Äù. ¬Ý
Gaining material insights
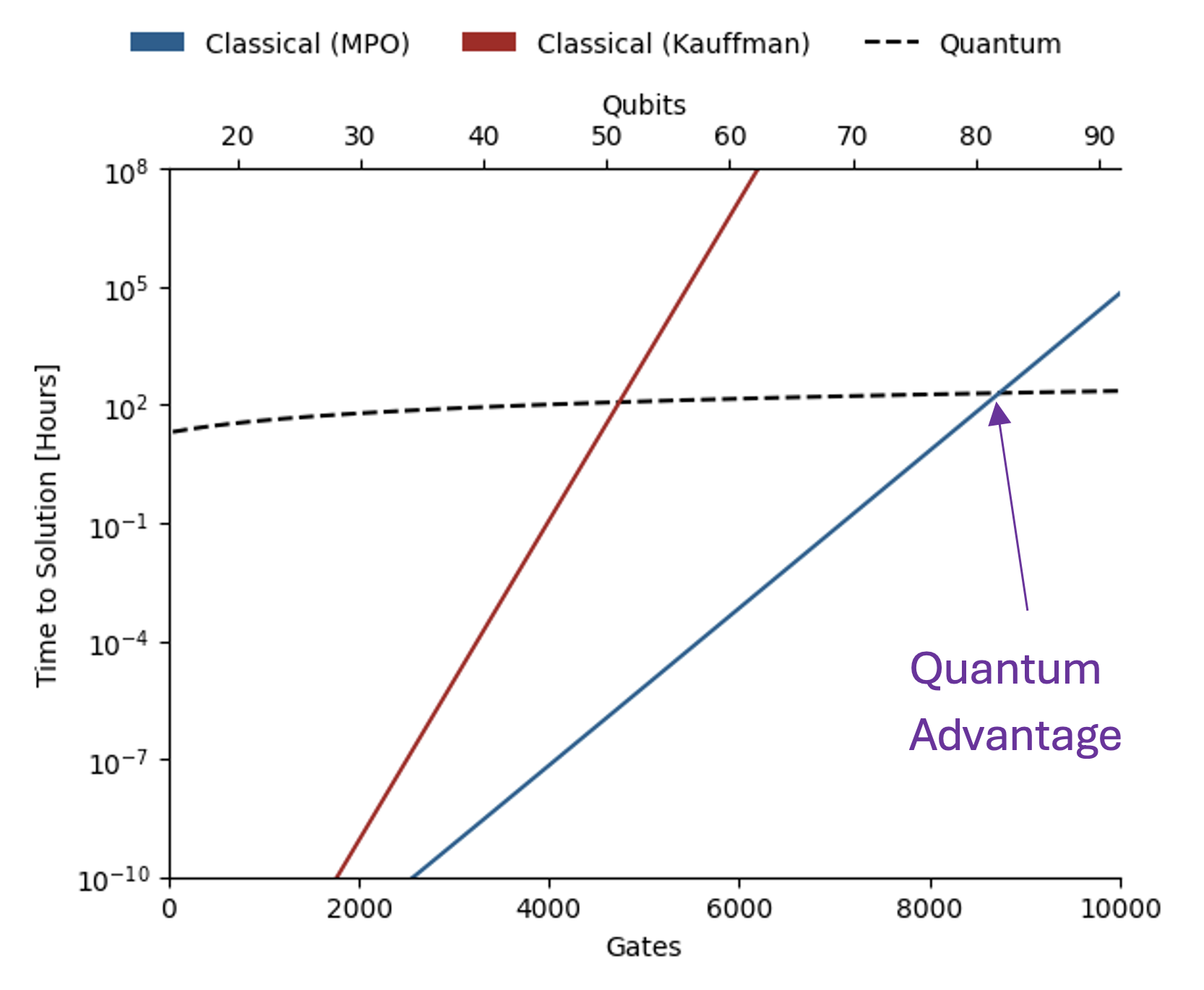
Researchers have struggled to simulate the dynamics of the Ising model at larger scales due to the enormous computational cost of doing so. Nobel laureate physicist Richard Feynman, who is widely considered to be the progenitor of quantum computing, once said, “.” When attempting to simulate quantum systems at comparable scales on classical computers, the computational demands can quickly become overwhelming. It is the inherent ‘quantumness’ of these problems that makes them so hard classically, and conversely, so well-suited for quantum computing.
These inherently quantum problems also lie at the heart of many complex and useful material properties. The quantum Ising model is an entry point to confront some of the deepest mysteries in the study of interacting quantum magnets. While rooted in fundamental physics, its relevance extends to wide-ranging commercial and defense applications, including medical test equipment, quantum sensors, and the study of exotic states of matter like superconductivity. ¬Ý
Instead of tailored demonstrations that claim ‘quantum advantage’ in contrived scenarios, our breakthroughs announced this week prove that we can tackle complex, meaningful scientific questions difficult for classical methods to address. In the work described in this paper, we have proved that quantum computing could be the gold standard for materials simulations. These developments are critical steps toward realizing the potential of quantum computers.
With only 56 qubits in our commercially available System Model H2, the most powerful quantum system in the world today, we are already testing the limits of classical methods, and in some cases, exceeding them. Later this year, we will introduce our massively more powerful 96-qubit Helios system - breaching the boundaries of what until recently was deemed possible.